Yearly Archives: 2022

Progressive Dice, a Misnomer

This is a follow-up article to “Progressive Dice for Effects Durations,” in which I propose a method to roll each turn for the chance for an effect to end. This, in order to maintain the secrecy—and suspense—of an effect’s duration when playing solo or otherwise without a DM.

So-Called “Progressive” Dice

“Instead of rolling for the effect duration at the trigger to know on what turn the effect ends, we roll the same dice at the beginning of each subsequent turn to see if the effect ends in that turn.

“A dice result equal to or greater than the current subsequent turn indicates the effect continues. Roll again at the beginning of the next turn. A lower result means the effect ends.”

—“Progressive Dice for Effects Durations”

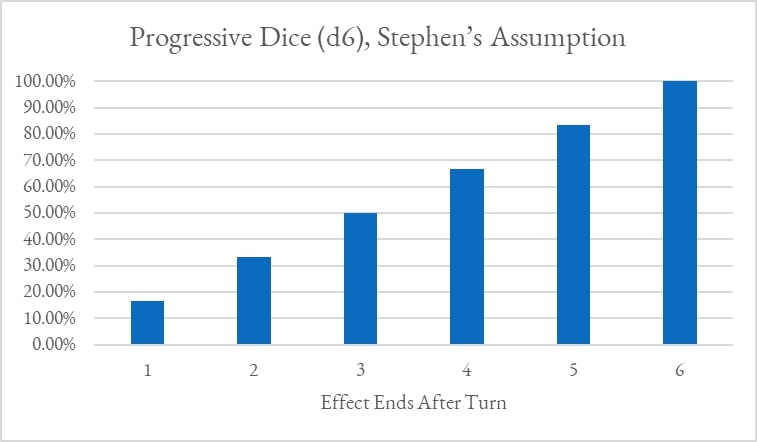
I’ve used progressive dice for effects durations and a number of other things for years. My assumption was that the chance for the effect to end each turn stands alone turn by turn, increasing as turns go by, therefore “progressive.” I also assumed that the overall probabilities, compared to the traditional method, were somehow the same.

Progressive Dice - Assumption

Writing the previous article forced me to think a little deeper on the method. I wondered if I’d got it right. Does it really yield a progressive chance, turn by turn, for the effect to end?

The smartest D&D mathematician I know is Dan Collins of Wandering DMs and Delta’s D&D Hotspot. A 40+ year D&D veteran, Dan is also a university lecturer in mathematics and computer science.

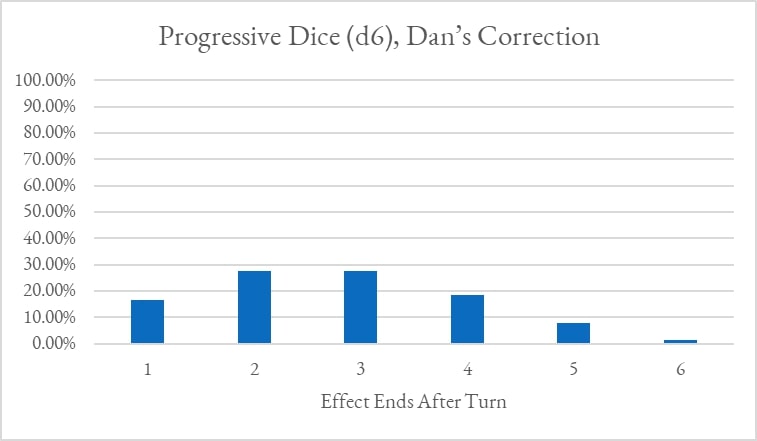
So, I sent him a query outlining the problem. Dan’s response, a few lines and a table of probabilities, shows how it is that progressive dice are not so progressive after all. For, using the proposed method, the chance of the effect ending is much higher in the initial periods than the later, so, not at all statistically equivalent to the traditional method.

In a traditional game, the DM rolls a single dice (or combination thereof) when an effect is triggered to determine its duration. A duration of 1 to 6 turns, say, is rolled on a d6. The probability that the effect ends on any turn is ⅙ or 16.67%.

Single Dice Roll [Traditional]

Using so-called progressive dice, “It stacks up differently,” Dan writes. “It’s very unlikely that you’ll get to turn 5 or 6, because you have to survive all the prior rolls to get there. Over half the time you’ll have the effect stop after two or three rounds.”

Here I had to make a saving throw vs. Death Ray. Reading the email, I was talking to Dan through the screen: The progressive dice method is so elegant, man—it has to be right!

Dan goes on to explain: “Computing a compound probability like this is a series of multiplications…” He also includes a table with a note that, if the calculations are correct, the sum of all chances should be 100%. I reproduce the table here.

Progressive Dice, Chance to End After Turn n
Turn Multipliers Product
1 1⁄6 16.67%
2 5⁄6 × 2⁄6 27.78%
3 5⁄6 × 4⁄6 × 3⁄6 27.78%
4 5⁄6 × 4⁄6 × 3⁄6 × 4⁄6 18.52%
5 5⁄6 × 4⁄6 × 3⁄6 × 2⁄6 × 5⁄6 7.72%
6 5⁄6 × 4⁄6 × 3⁄6 × 2⁄6 × 1⁄6 × 6⁄6 1.54%
Total   100%

So, it isn’t just the simple chance (bold) each turn that the effect will end. We have also to factor in the cumulative chance (italics), which is each previous roll inverted, that the effect hasn’t already ended.

Note that, in the previous article, we roll to see if the effect ends at the beginning of the next turn. “Ends after turn n” is a different way to say the same thing.

Progressive Dice - Correction

Therefore, at best, I misnamed “progressive dice.” Though the number to roll increases turn by turn, the chance to make that number is not at all progressive. The chance to end the effect after the second or third turn is much higher than the first or later turns.

Alternatives

So, what is a DM-less player to do? We might accept the statistical difference and use the so-called “progressive” dice in play. Or we might seek out other solutions. We look here at two—one of them works.

Single Dice, Effect Ends on a 1 (Not a Solution)

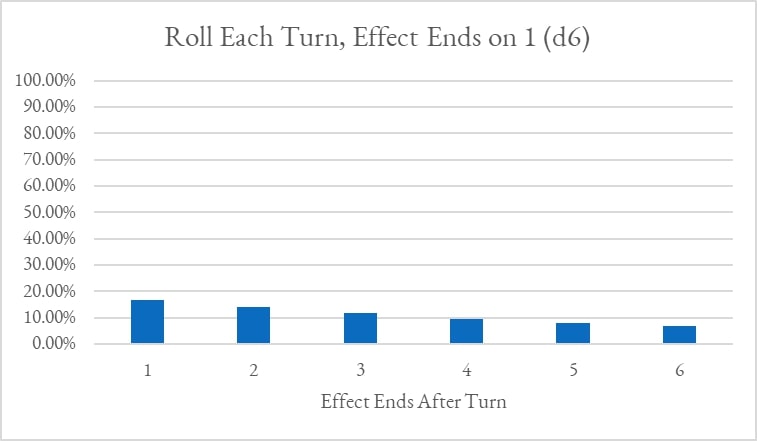
I thought of an alternative method. Roll the same dice every turn, with a result of 1 signaling the effect’s end. The effect ends automatically at the end of the  maximum duration.

It’s more simple than counting turns. But, if I’m following Dan’s lesson well, we still have to factor in the chance that the effect ended with the previous roll(s).

Effect Ends on 1, Chance to End After Turn n
Turn Multipliers Product
1 1⁄6 16.67%
2 5⁄6 × 1⁄6 13.80%
3 5⁄6 × 5⁄6 × 1⁄6 11.57%
4 5⁄6 × 5⁄6 × 5⁄6 × 1⁄6 9.65%
5 5⁄6 × 5⁄6 × 5⁄6 × 5⁄6 × 1⁄6 8.04%
6 5⁄6 × 5⁄6 × 5⁄6 × 5⁄6 × 5⁄6 × 1⁄6 6.70%
Total   66.51%

Ends on 1

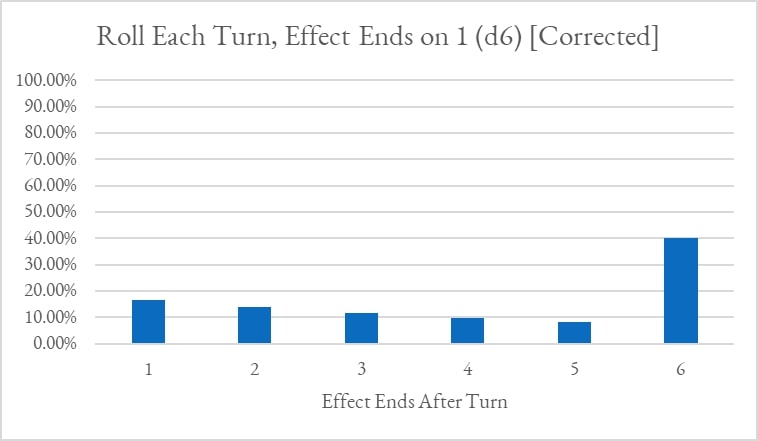
Furthermore, I note that the total percentage is only 66.51, which is 33.49 short of 100. I’m guessing that’s because the effect automatically ends at the duration’s upper limit. The chance that it will end after the 6th turn is, in fact, 6.70 plus the remaining 33.49, or 40.19%.

Ends on 1 - Corrected

1 to n Cards

Dan suggests a card solution: a number of playing cards n equal to the upper limit of the range, 1 to n, one of which is an ace—or, if you have a deck of many things on hand, the Donjon (ace of spades) or the Fates (ace of hearts).

Shuffle the deck once when the effect is triggered. Draw one card from the top of the deck at the beginning of each turn. When the ace comes up, the effect ends.

Here, the shuffling is the dice roll, which determines on which turn the effect ends (on the ace). The chance that it will end in any particular round is 1⁄n, just like a single dice roll. The only practical difference from the dice roll is that the ending turn, while predetermined, is hidden within the deck. Also elegant.

A disadvantage is that the card method cannot duplicate dice combinations. Melqart’s stun duration, 2d4 turns, for example, cannot be reproduced using this method. In this case, it was the first effect duration of the campaign, but dice combinations might be infrequent.

Another disadvantage is that you have to manage an additional tool at the table. The suspense about when the effect ends, though, may well be worth the trouble.

For myself, I love to incorporate playing cards into my D&D, and if there’s an opportunity to get more use out of a deck of many things, I’ll take it.

Other Solutions?

I’m interested to hear your suggestions for maintaining the secrecy of effects durations in a DM-less game. I would also entertain a counterargument showing that progressive dice do in fact produce progressive results. Because it’s elegant, man, it has to be right!

My thanks to Dan Collins for his statistical analysis of the problem as well as an alternative solution. For interested readers, Dan offers several venues to learn more about dice and probability. In an episode of Wandering DMs, Dan gives a course in Basic Dice Math, and in another episode with cohort Paul Siegel, he talks Dice Mechanics. In addition, you’ll find a plethora of articles about dice statistics on Dan’s blog.

Player Character Record Sheet Index Cards

Tabletop Playing BX D&D

 
Real estate is valuable at one’s place on the table. A solo player needs, within easy reach, maps, notebook, rulebook, setting guide, and adventure notes, in addition to dice and a place to set a drink. Small room remains for a character sheet, less for a whole party of them.

Years ago, I started with full-page character sheets but soon reduced to half size, before I realized the utility of the 3" × 5" card.

Thorsdottir BX Player Character Record Card Front Thorsdottir BX Player Character Record Card Back
B/X Player Character Record Card, Front and Back.

Compared to the official B/X accessory (reproduced B14),1 the index card lacks saving throw and “to hit” vs. AC tables, notes, character sketch, and player’s name.

The D&D Reference Tables (from Dungeon Module B2, “perforated for easy removal”) replace the save and “to hit” tables. I keep notes in the adventure log, player’s name is omitted for solo games, and my drawing skill does nothing to improve the record’s aspect.

The index card is adaptable to other early D&D editions. For Endys the Uncanny, created when graph paper was not available to me, I use a character card in a Holmes D&D Basic game. Images below show the card of Palantir, an OD&D character.

Palantir OD&D Player Character Record Card Front Palantir OD&D Player Character Record Card Back
OD&D Player Character Record Card, Front and Back.

The OD&D card includes space for the character’s class, “Elf Fighting Man,” rank, “Veteran,” and Fighting Capability, “HF/AF.” The reverse has room to note a Beneficiary, in this case, a nephew Fingolfain.

The field for damage can also be used to note the manner of the character’s passing. Palantir was killed by a ghoul in room 9 of some deep, dark place. Should Fingolfain seek the inheritance, he may find his uncle’s gnawed bones in an open tomb. We note, however, Palantir, in his short career, gained not a gold piece.

Palantir in Play Prior to His Demise in an OD&D Campaign
Palantir in Play Prior to His Demise in an OD&D Campaign.

Notes

1 These sheets for B/X were produced from 1980 through 1984.

Cover Player Character Record Sheets 1980 Cover AC 5 Player Character Record Sheets 1984
D&D Player Character Record Sheets.
Covers by Jim Roslof (TSR, 1980) and Clyde Caldwell (TSR, 1984).

“A Dagger For Protection”

“Magic-users — humans who elect to become magic-users must not wear armor and can carry only a dagger for protection” (Holmes, 6).

Reading Holmes on a Sunday morning—as one does—gave me an idea for a magic item.

Though we might say one carries a weapon “for protection,” it really doesn’t protect us so much as it should harm an aggressor. I thought, what if…?

New Magic Item

Dagger of Protection — as a dagger +1 in combat. It is paired with a steel sheath. Only while sheathed does the dagger protect the carrier, adding +1 to armor class and saving throws. Also called a “mageblade.”

OD&D’s “Recommended Equipment”

A 1st- to 9th-Level Campaign
The Outdoor Survival Map Amid Other Tools for a D&D Campaign.

Don’t Throw Out the Box the Map Board Came In

In this article, I don’t mean to say anyone is playing the game wrong. I mean to say that our OD&D games—or at least our esteem of the rules—might improve if we reconsider the ignored parts of Chainmail and Outdoor Survival.

A recent Grognardia article reminds me of a point I’d like to bring up. In “Retrospective: Outdoor Survival,” James Maliszewski gives adequate treatment to the 1972 simulation game, with due attention to designer Jim Dunnigan, mention of the included Wilderness Skills primer—which reminds James of The Boy Scout Handbook, and a brief summary of play and the five scenarios typical of a wilderness environment: Lost, Survival, Search, Rescue, and Pursue.

He doesn’t miss the map board, of course, and its suggested use in OD&D as the setting for impromptu adventures. James notes that Outdoor Survival is the second entry in Vol. I under the heading “Recommended Equipment.”

When playing OD&D, I think1 we don’t take the rulebook’s advice seriously enough. It’s true, “Recommended Equipment” is misleading. Considering the “Dungeons and Dragons” rules are first in the list, “Required Equipment” would be more accurate. We would hardly think of playing D&D without dice, to cite the list’s third entry.

Likely due to the cost of two more games in addition to the ten 1970s dollars we already spent on a box of three slim booklets—not to mention dice, we content ourselves to replace Chainmail with the Alternative Combat System and sometimes use Outdoor Survival’s map board as a wilderness setting.

In so doing, we neglect the other—admittedly cumbersome—combat rules, like move-and-countermove (Chainmail, 9), parry and number of attacks per round by weapon class (25-26), and I’ve talked enough about jousting.2 In fact, the Alternative Combat “System” replaces, with a d20, only Chainmail’s fistful of dice to determine hits.

Later D&D editions revisited Chainmail to restore some of the combat options. The Holmes edition’s oft-bemoaned implementations of parry and number of attacks per round (20-21) are examples, as is B/X’s oft-ignored combat sequence (B24). But OD&D combat, bereft of these options, becomes the stereotype “I miss, I hit… I miss again.”

We also explore the wilderness on a hex map, but without any dangers apart from monsters with lots of hit dice rolled on the Wilderness Wandering Monsters tables. For this reason, commenter Gus L., in response to James’s article, likens adventures in the OD&D wilderness to “a bus ride with fistfights.”

It doesn’t have to be that way. On the page before the wilderness monster table, Vol. III refers us to Outdoor Survival’s rules as well as its board to handle lost parties (17). Further, when a party becomes lost, food may well run short. In a desert, water is scarce. Maybe it makes for a less than heroic adventure, but rules to handle starvation, thirst, weather, and fatigue are found in Outdoor Survival. By breaking up the succession of fistfights, incorporation of those rules can turn the bus ride into a challenging journey accompanied by the threat of many-hit-dice monsters.

Grognardia doesn’t mention Outdoor Survival’s most interesting innovation for an early 1970s game. After we’ve learned the rules playing a Lost scenario and maybe a Search or a Rescue, lackluster as they may be, we must press on to Scenario 6.

Scenario 6: One of the most interesting aspects of OUTDOOR SURVIVAL is the opportunity it provides for devising your own scenarios. Once you have mastered the mechanics of play, many additional ideas, providing more testing of outdoor knowledge and skills, will come to you. Integrating these situations with the standard games will add pleasure and skill-sharpening to the playing.

—Jim Dunnigan, Outdoor Survival

There is a certain irony in that Scenario 6 appears under the heading “Optional Rules.” For best results, I recommend using “Dungeons and Dragons”—those slim booklets containing lists of spells and monsters—as additional equipment.

Notes

1 I use “I think” as a lazy and weak shield against attacks from those whose opinions differ. Excuses to my sophomore English composition teacher, who pointed out, “If you didn’t think it you wouldn’t write it, would you.”

2 Strategy on the Jousting Matrix

Turn Undead in Movement Phase

It was a table perhaps some decades ago. Remaining now are crumbling bits of dry rot wood next to a single stool in similar condition. One plate, flatware, a goblet, and two candlesticks, all of tarnished silver, lay amid the friable refuse.

Bending, Hreidmar scooped up the goblet. “Now there’s a treas—”

The north door opened and a troop of skeletons filed in. The first held a covered plater high in one hand, a threadbare towel laid over the bones of the other arm.

Thorsdottir stepped forward and thrust the Ouroboros1 toward the advancing column—“Back!”

The silver platter dropped to the floor with a clang. The cover rolled aside. Heel bones scraped stone as the skeletons turned away, fleeing through the door…

Lower Levels of the Lonely Tower
Lower Levels of the Lonely Tower.
The scene takes place in the central room (unnumbered), lowest level.

I’ve always counted Turn Undead as some kind of magic for the purpose of when, in the combat sequence (B24), a cleric should take the action. The B/X Rulebooks give no guidance on the matter (nor on a number of other details about Turning).

In my experience, Turning in the magic phase makes for some awkward moments:

  • Maneuvering and preparation for combat against half a dozen or so undead takes up time that will be for naught if the cleric’s Turning is successful.
  • Players, thinking about tactics in the movement phase, make a certain emotional investment in the combat, of which they are then deprived.
  • Missiles fired on the undead before they are Turned in the following phase is often anticlimactic.

During a recent Wyrmwyrd session, it occurred to me that Turning is only a quick gesture and maybe a couple spoken words. A cleric could easily do that while moving. If successful, the field is cleared—or at least thinned. If the Turning fails, the players can get into the combat with confidence their actions will be meaningful.

Rules Clarification: Turn Undead

A cleric attempts to Turn Undead in the movement phase of the combat sequence (step B, phase 2).

In the scene depicted above, neither side was surprised, and the player party won the initiative roll. Thorsdottir turned seven of nine skeletons.

Notes

1 The Ouroboros is the holy symbol of the Pantheon.

A Cleric Presents a Holy Symbol

 

The Blackmoor Finger

“The basic campaign area… was originally drawn from some old Dutch maps.”

—Dave Arneson, First Fantasy Campaign, 11

Like many Blackmoor fans, I’ve often wondered about—and long sought—these “old Dutch maps.” It isn’t surprising that the naval wargamer would have on hand maps made by one of the great maritime powers of the Age of Sail. But would these Dutch maps depict the homeland or some distant discovery, like the Falklands or the Hudson Valley?1, 2

An Old Dutch Map

 

Yet, Blackmoor has in common with the Low Countries at least its soggy biome. In 2009, James Mishler of Adventures in Gaming took a 1520 map of Holland, flipped it over vertically, and rotated it 90 degrees to the left.3 He invites us to compare the results to the earliest then-known sketch map of Blackmoor, which is found in First Fantasy Campaign (12).

Mishler also notes that the image manipulation places any page footer text to the left of the map, that is, westward. Apocryphal or no, the legend goes that the Duchy of Ten, west of Lake Gloomy, is named after a “10” on the map. This, as Mishler postulates, might mean we should be looking for a map from a book that uses intaglio plates, the reverse side of which are blank.

Map of Holland - Flipped and Rotated Blackmoor Sketch - First Fantasy Campaign
Map of Holland, Flipped and Rotated, and Blackmoor Sketch, First Fantasy Campaign.
Frankly, I can make the Blackmoor sketch look like just about anywhere if I squint just right.

To wrap up a multi-part review of First Fantasy Campaign, Bat in the Attic Rob Conley searched out an old Dutch map to dress up as a fantasy realm. But, he writes, “I couldn't quite figure out which old Dutch map to use or how it looked anything like Blackmoor.” Finally, Conley used Mishler’s method to create the map for his Blackmarsh old-school setting supplement.4

Rob Conley’s Blackmarsh
Rob Conley’s Blackmarsh.

Not satisfied with the results obtained from the Mishler method, I continued the search for “old Dutch maps.” As often happens during these rabbit-hole explorations, I learned lots of things—one of many reasons D&D is a superior game.

Having heard the legend of the little Dutch boy who plugged with his thumb a leaking dike, most of us are aware that the Netherlands has been sinking into the North Sea for centuries. Since the middle ages, the inhabitants have drained lakes to reclaim land, and an extensive engineering project called the Zuiderzee Works, in the 20th century, damned the central bay and created a number of polders.5

The practical upshot of all this is that not all old Dutch maps look the same. As man struggles against nature, land creeps above the waterline then slips below, year by year, as though subjected to the crests and troughs of a long-period tide.

Since Mishler’s go at matching Blackmoor to Holland, an earlier Blackmoor map surfaced. Most online references point to an article on Secrets of Blackmoor, which shows a colored version of what it calls the “Original Blackmoor Map.” The map is accompanied by a one-page document describing a “medieval project.”6 The document’s first enumeration reads as follows:

“See the enclosed map which represents the area known as the Northern Marches which guard the frontier of the great Empire of Geneva from the ever present threats presented by those who lurk beyond the light of our great empire and its great king.”

Blackmoor Facts - Hoyt Blackmoor Map - Hoyt
Document and Map Concerning the Northern Marches, Courtesy William Hoyt.

Stamps (not shown above), presumably placed by Secrets of Blackmoor, on both document and map credit the source as Twin City gamer and Arneson contemporary William Hoyt. The document’s text concludes with the initials “D.A.”

OSR Grimoire compares this earlier map to Mishler’s 1520 Holland map without alteration.

Original Blackmoor Map Compared to a Holland Map
“Original Blackmoor Map” Compared to a Holland Map.

The U-shaped coastline matches up fairly well, the extreme west coast, though short, matches better, the Dutch location looks fearsome enough to accommodate the Egg of Coot, and a few Dutch roads share a similar angle as some Blackmoor waterways.

Still, though, I’m not convinced. The search continued until I saw the finger.

A salient feature of the earlier Blackmoor map, reproduced on the foldout map in First Fantasy Campaign, is a strip of land that extends from the Glendower peninsula, pointing northwest toward the Egg of Coot. I always thought Dave must be giving someone the finger—I don’t know who…

Map of the Republic of the Seven United Netherlands Janssonius 1658
Map of the Republic of the Seven United Netherlands, Janssonius, 1658.

This 1658 Map of the Republic of the Seven United Netherlands7 (above) shows a similar land mass, perhaps a tidal flat (upper middle). Considering the fist behind, it is pointed the opposite direction. Applying the Mishler method, however, orients the finger to align with that of the earlier Blackmoor map. The fist becomes the Glendower peninsula.

Earlier Blackmoor Map Overlay on Janssonius 1658 Map
Earlier Blackmoor Map Overlay on Janssonius 1658 Map.

To align the finger just right, I rotated the Blackmoor map 10.29 degrees. I note also that, on importing the Blackmoor map, I didn’t have to change the scale. Coincidence, perhaps.

Earlier Blackmoor Map Transparent Overlay on Janssonius 1658 Map Cropped to Blackmoor Area
Earlier Blackmoor Map Transparent Overlay on Janssonius 1658 Map, Cropped to Blackmoor Area.

The finger of land aligns well, but nothing else matches. Maybe the Blackmoor map is an amalgam of different takes on the same or several maps.

I am yet far from convinced. But a coincidence of geography seems unlikely. Arneson may well have traced features on the reverse of a “Plate 10.” Future searches for “old Dutch maps” might keep an eye out for those showing the Blackmoor finger.

Notes

1 A thread on the OD&D Discussion forum bounces around the idea that Arneson may have been inspired by the Minnesota coast of Lake Superior.

2 Browse a few dozen old Dutch maps on Stanford’s Renaissance Exploration Map Collection.

3 Mishler’s article “Original Blackmoor Maps,” no longer available at the original address, is now found at Adventures in Gaming v2 as “[Throwback Someday Post] Original Blackmoor Maps.”

4 Blackmarsh: A Setting Supplement Compatible with the Delving Deeper Rules and All Editions Based on the Original 1974 Roleplaying Game, Robert Conley, 2011. Available in print or PDF on DriveThruRPG.

5 Polders: My untrained eye spots some of these areas of reclaimed land on a satellite image.

6 In response to a question in the comments to the Secrets of Blackmoor article, Griff Morgan writes that several pairs of the map and document exist.

7 Wikimedia Commons identifies Joannes Janssonius as the cartographer of the Map of the Republic of the Seven United Netherlands from the collection Belgii Foederati Nova Descriptio, Amsterdam, 1658.

Secret Room Under Landing

After helping to find a secret door below the entry landing, Hreidmar and company fell into the order of march behind Thorsdottir, Gandrefr, and Ardur.

Entry Level and Secret Room Under Landing
Entry Level and Secret Room Under Landing.

Secret Room

A deep step down leads into this small room. The ceiling, below the landing above, is only six feet high. A door on the left (north) is closed.

Opposite the step (southeast) is a wide alcove, the back wall of which is tiled with lavender-tinted crystal. On a ledge stands a statuette, blackened by age. The figure is male, head turned toward an outstretched arm. The arm, as well as the statuette’s base, is truncated in a rounded mass, as if previously melted.

When Thorsdottir reached for the statuette, a bright flash of light from the crystal tiles left her blinded. Ardur and Hreidmar were also effected.

The other dwarves entered the room, letting the secret door close behind them. The four guarded the two entry points in pairs, while the party waited for their comrades’ sight to be restored.

Gandrefr examined the statuette, which she took from Thorsdottir’s hands. Scratching the black surface, she said, “The statue is of bronze. It’s damaged but should be worth something.” She stowed it in Thorsdottir’s pack.

Not having playing cards on hand to determine the effect’s duration, which was 1 to 8 turns, I used a deck of Chainmail spells I made last summer, Wizard Light as the ace. The party waited 40 minutes for sight to return.

At the table, the time passed quickly. I drew a card at the beginning of each turn and checked for wandering monsters at the end of every other. There was one: wolves.

I didn’t see how wolves could open either the secret or the normal door, which “usually open automatically for monsters” (B21). So…

The party waited, cramped in the small space, heads bent beneath the low ceiling. Then a scratching sound, as claws on wood, broke the silence. The dwarf guards braced their shields. The blind shrank back into the alcove.

Again the scratching, then “Awooo…” The canine howl came, not from an adjacent space, but from above.

Gandrefr let out a breath. “It isn’t here,” she whispered. “It’s at the entrance, just above.”

More scratching at the door before silence invested the space again.

“I can see,” said Thorsdottir, peering at shadows on the ceiling. She judged the distance from the secret door to the alcove. “This alcove must be directly below the arched door.”

Gandrefr recalled the archway’s inscription: “Lost alone together found…”

Though the DM suspects that the damaged statuette and the archway inscription have some connection, he does not yet know what it is.